Percentage Concepts, Formulas, Calculation Tricks, Shortcuts and Examples

---

In any exam in which Quantitative Aptitude is asked, the topic ‘Percentage’ is the most important topic. The topic Percentage has its application in almost all the topics in the arithmetic section, e.g., Profit and loss, Mixture and Alligation, Simple Interest and Compound Interest, Time and Work, Time, speed and distance. Also, Percentage is very important in the purview of calculations. It plays a vital role in Data Interpretation questions, as most of the questions asked are based on Percentages.

So, let us understand the basics of Percentage and see how questions are asked from this topic.

Definition:

The percent is derived from the Latin word ‘Centum’ which means for ‘every 100’ or ‘per hundredth.’

“Percentage is the representation of a number, ratio, fraction, or decimal as a fraction of 100.”

Representing number, fraction, ratio and decimal in the form of Percentage

1. Number

To represent a number ‘N’ into Percentage, just simply multiply the number with 100.

Example:  Convert ‘4’ into Percentage.

Solution: Representation of 4 in Percentage will be

= 4 × 100 = 400%

2. Fraction

To represent a fraction into Percentage, just simply multiply the fraction with 100 and to convert Percentage into fraction just simply divide the Percentage by 100.

Example: Convert the fraction \(\dfrac{3}{5}\) into Percentage

Solution: Representation of \(\dfrac{3}{5}\) into Percentage will be

=\(\dfrac{a}{b} *100=60 \%\) 

3. Ratio

To convert the ratio into Percentage, first convert the ratio into a fraction and then multiply the fraction with 100.

Example: Convert the ratio 2 : 5 into Percentage?

Solution: Converting ratio into fraction we get

\(2:5 \implies \dfrac{2}{5}\)

Now, the representation of \(\dfrac{2}{5}\) into Percentage will be

=\(\dfrac{2}{5}×100=40 \%\)

4. Decimal

To represent a decimal into Percentage just simply multiply the decimal with 100 and to convert Percentage into decimal just simply divide the Percentage by 100.

Example: Convert 0.773 into percentage

Solution: Representation of 0.773 into percentage will be

=\( 0.773 × 100 = 77.3 \%\)

To solve the questions based on Percentage, one should learn some important conversion of Percentages into Fractions.

Let us represent some fractions in terms of Percentage in the tabular form for you

Some other important fraction values in terms of Percentage are

NOTE: learning all the important fractions will save you a lot of Time.

1. If there is 0.11, 022, ……., 0.88, and 0.99 in the Percentage, then the fraction corresponding to the Percentage will have 9 or multiple of 9 in the denominator.
2. If there is 0.33 and 0.66 in the Percentage, then the fraction corresponding to the Percentage will have 3 or multiple of 3 in the denominator.
3. If there is 0.1428, 0.2856, 0.4285, 0.5713, 0.7142 and 0.8571 in the Percentage, then the fraction corresponding to the Percentage will have 7 or multiple of 7 in the denominator.

Increase/Decrease in Percentage

Percentage increase/decrease is the ratio of the change in number/quantity with respect to the original number/quantity.

 

1. When A is increased by x%, then a new number B is obtained. To obtain the number A again, we have to decrease the number B by

\(\left(\LARGE \dfrac {x}{100+x} \right)×100 \%\)

2. When A is decreased by y%, then a new number B is obtained. To obtain the number A again, we have to increase the number B by

Let us understand the above concept through questions

Example: Raghu has 20% more toffee than Pinki. How much percent less toffees Pinki have as compared to Raghu?

Solution:

Here, the quantity of toffees with Raghu is 20% more than Pinki, i.e., the value of x is 20%

So, Percentage of toffees with Pinki as compared to Raghu will be less by:

\(\implies \left(\dfrac {x}{100+x} \right)×100 \%\)

Alternative:

Let us assume toffees with Pinki be 5 units

So, toffee with Raghu will be 6 units

\( =\dfrac{1}{6}*100=16.66 \%\)

Note: When ‘percentage increased/decreased by X%’ is given for a quantity, then the actual increase/decrease in the quantity is (\(100 ± X\)%)

While when ‘percentage increase/decrease to X%’ is given for a quantity, then the actual increase/decrease in the quantity is (\(±X\)%)

For example, if the salary of a person is decreased by 20%, then the final salary of the person will be 80% of the original salary while if the salary of a person is decreased to 20% of the final salary of the person will be 20% of the original salary.

Concept of Product constancy

Product constancy involves the problems with two dependent quantities whose product is the third quantity. Thus, if there is an increase in the first quantity, then to make the third quantity constant, we have to increase/decrease the second quantity.

NOTE:

If there is an inverse relationship between the first and second quantity, then on the increase of the first quantity, we have to decrease the second quantity and vice versa.

• If there is an increase of x% in first quantity, then for the third quantity to remain constant, we have to decrease the second quantity by a factor

\(\left(\LARGE \dfrac {x}{100+x} \right)×100 \%\)

• If there is a decrease of y% in first quantity, then for the third quantity to remain constant, we have to increase the second quantity by a factor

\(\left(\LARGE \dfrac {y}{100-y} \right)×100 \%\)

If there is a direct relationship between first and second quantity, then on the increase/decrease of first quantity by x%, the second quantity will be increased/decreased by x%.

Some relations from which questions on product constancy are asked are:

1. Expenditure = Price * Consumption
2. Price = Rate * Quantity
3. Distance = Speed * Time
4. Work done = Time taken * Efficiency
5. Area of Rectangle = Length * Breadth

Example: The price of sugar is increased by 25%. If a family does not want to increase/decrease their expenditure, then by what percent should it decrease their consumption?

Solution: We know, Expenditure = Price * Consumption

As, price is increased by 25%

So, here x = 25%

Thus, to remain the expenditure constant we should decrease the consumption by

\(\implies \left(\dfrac {x}{100+x} \right)×100 \%\)

Quantities having relation in the form of C = A + B

In three quantities having a relation of C = A + B, quantity A is increased by x%. If we wish to maintain C as constant, then the B should be reduced by \(=(x \space of \space \dfrac{A}{B}) \%\) and vice versa in case of a decrease.

Example: The saving of a person is 20% of his income. If his expenditure increases by 10% without any increase in his income, then by how much percent he has to decrease his saving?

Solution:

Let us assume income of the person be 100

So, initial savings of the person = 20% of 100 = 20

We know, Income = Expenditure + Saving

Expenditure of person = 100 – 20 = 80

According to the question,

Final expenditure of person = 110% of 80 = 88

As, income is constant so final income = 100

Final Saving = 100 – 88 = 12

Alternative Approach

Here, the increase in expenditure is 10%

So, \({\small X }= 10\%\)

\(\implies \left(\ 10* \Bigg( \dfrac {80X}{20X} \Bigg)\right)\%=40\%\)

Successive Percentage increase/decrease

If a number N undergoes a successive percentage increase/decrease of a%, b%, c% and so on then after a net change in the number, the final value of number will be

Application of Percentage on Population increase/decrease

If there is an r% increase/decrease in population of a place in a year, then

The Population of a place n years later will be

\(=P \Big(1± \dfrac{r}{100} \Big)^n\)

And, population of a place n years ago will be

\(=\dfrac{P} { \Big(1± \dfrac{r}{100} \Big)^n}\)

Type of questions asked from Percentage

Type 1: If \(\dfrac{5}{9}\) is multiplied instead of \(\dfrac{2}{3}\)in a number, then what will be the percentage error in the calculation?

Solution: Let the number be LCM of 9 and 3 = 9

\(Now, \space Error \space number=\dfrac{5}{ 9}*9=5\)

\(Correct \space number=\dfrac{2}{3}*9=6\)

\(\implies \dfrac{6-5}{6}*100=16.66 \%\)

Type 2: The population of a town increases by 20% each year. If the population of the town 3 years ago was 2500, then what is the present population of the town?

Solution: Let us assume the present population of the town is P

We know,

The population of a place n years ago will be

\(=P \Big(1± \dfrac{r}{100} \Big)^n\)

According to the question,

\(\dfrac{P} { \Big(1+ \dfrac{20}{100} \Big)^3}=2500\)

\(\dfrac{125P}{216}=2500\)

\(P = 4320\)

Thus, the present population of the town is 4320.

Type 3: In an election between two candidates, 10% of the voters didn’t vote. 10% of valid votes are declared invalid. A candidate gets 210 votes more than the other candidate, who got 44% of the total votes. What is the total population of voters in the constituency?

Solution: Let us assume total population be 100X

According to the question,

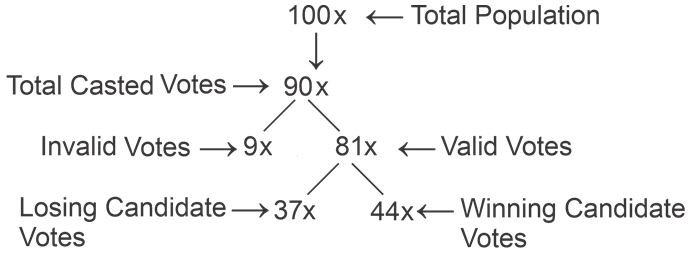
From the above figure we can say that

Difference between votes obtained by winning candidate and that by losing candidate will be

= 44X – 37X = 7X

According to the question,

7X = 210

X = 30

Therefore, total population = 100 * 30 = 3000

Type 4: In an examination, out of 400 students, 54% of boys and 70% of girls pass. If the total pass percentage was 60%. Find the total number of girls.

Solution: Let the number of girls be x

Thus, number of boys will be 400 – x

According to the question

Number of boys who pass = 54% of (400 – x)

Number of girls who pass = 70% of x

Total number of students who pass = 60% of 400

Thus,

60% of 400 = 54% of (400 – x) + 70% of x

240 = 216 – 0.54x + 07x

0.16x = 24

x = 150

Type 5: If A earns 20% more than B, B earns 25% more than C, C earns 16.67% less than D, then A earns how much percent of D?

Solution: We know,

According to the question,

\(A=\dfrac{6}{5}B\)

\(B=\dfrac{5}{4}C\)

\(C=\dfrac{5}{6}D\)

Combining all the above equation,

\(A=\dfrac{6}{5}*\dfrac{5}{4}*\dfrac{5}{6}D\)

\(\implies A=\dfrac{5}{4}D\)

We know, \({5 \over 4}=125 \%\)

So, we can say that A is 125% of D.

Type 6: A salesman gets a 10% commission on the sales up to ₹ 25,000. On sales above ₹ 25,000, he gets a total commission of 15%. If he deposited a total sum of ₹ 86,250 in the company, then what will be the total earnings of the salesman?

Solution: If the company would have given 15% of commission instead of 10% on the sale up to ₹ 25000 also, then the company would receive some less amount which will be equal to

Loss of company = 5% of 25000 = ₹ 1250

The amount received by company = ₹ 86250

If the salesman would have given 15% of commission on all the sales, the amount received by the company will be

= ₹ 86250 - ₹1250 = ₹ 85000

We know,

\(15\%=\dfrac{3}{20}\)

Thus, on the sale of 20 units, he will earn 3 units

So, earnings of company will be = 20 – 3 = 17 units

Here, 17 units correspond to ₹ 85000

So, 1 unit will correspond to ₹ 5000

Now, earning of a salesman if he would have given 15% of the commission will be = 3 * 5000 = ₹15000

But, in actual he has been given only 10% commission for starting ₹ 25,000

So, we can say that the actual earning of salesman = ₹ 15000 – ₹ 1250 = ₹13750

Share Blog