QOTD | Quantitative Aptitude | Mensuration | Surface Area and Volume

Question of The Day15-11-2019

A cone of height 16 cm into two parts by a line parallel to the base of the cone such that a smaller cone of height 4 cm and a frustum are obtained. What will be the ratio of the curved surface area of the frustum and the larger cone?

Answer

Correct Answer : c ) 15 : 16

Explanation :

Let us assume the height, radius and slant height of a smaller cone are h, r, and l respectively

Let us assume the height, radius and slant height of a larger cone are H, R and L respectively

Depicting the above information in the figure we get,

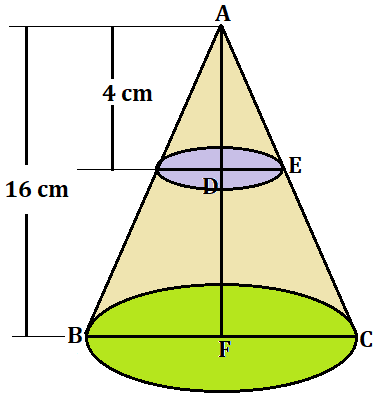
Quantitative Aptitude Mensuration Surface Area and Volume QOTD - 15 November 2019

From the above figure,

In triangle ADE and triangle AFC

∠DAE = ∠FAC (common angle)

∠ADE = ∠AFC = 90⁰

Therefore from Angle – Angle (AA) property we can say that ▲ADC ≅ ▲AEC

Thus,

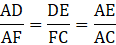
Therefore,

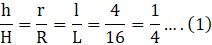
As we know that

The curved surface area of the smaller cone (CSA)_S = πrl ….. (2)

The curved surface area of larger cone (CSA)_L = πRL …… (3)

Dividing equation 3 by 2 we get

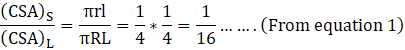
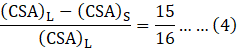
As Curved surface area of Frustum (CSA)_F will be = (CSA)_L – (CSA)_S ….. (5)

By comparing equation 4 and 5 we get

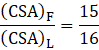
Hence, (c) is the correct answer.

Such type of question is asked in exams like SSC CGL, SSC MTS, SSC CPO, SSC CHSL, RRB JE, RRB NTPC, RRB GROUP D, RRB OFFICER SCALE-I, IBPS PO, IBPS SO, RRB Office Assistant, IBPS Clerk, RBI Assistant, IBPS RRB OFFICER SCALE 2&3, etc. Try and attempt free mock tests at PendulumEdu. Read Daily Current Affairs and Banking Awareness to improve your preparation level for the government exams.